Quantum Information Processing
Trapped ions were arguably the first experimental platform to be used as quantum information processors and for almost 25 years our group has worked at the foundations of this now sprawling field. In this quarter century the group has grown steadily and now hosts several information processors with different focuses, register sizes, and form factors. Along with this growth in size and number of processors comes the breadth of principal research directions. These research topics include:
- Quantum error correction and fault tolerance - How can we make quantum computation tolerant to errors?
- Quantum characterisation, verification and validation - How can we make sure the output of a quantum computation is trustworthy?
- Qudits as higher-dimensional quantum information carriers – How can we make good use of quantum information carriers beyond limiting ourselves to two levels (qubits)?
- Scalable quantum information processor development – How do we build ever larger quantum computers and how to we operate and maintain them?
Over the years our research has been graciously supported by a great many projects, including:
- The EU programmes AQTION, MILLENION, PIEDMONS
- World-wide collaborations such as LogiQ, QCPR, and LASCEM
- National excellence funding such as the FWF SFB BeyondC
An ion trap-based quantum computer
In our experiments we make use of a combination of static and dynamic electric fields to trap and cool singly-charged atoms (ions) of Calcium-40 in ultra-high vacuum. Upon laser cooling these ions freeze into stationary structures called ‘ion crystals’ that behave much like a solid. We most frequently shape these crystals into strings in what has since come to be known as the linear Paul trap. These strings form the registers which we use for quantum information processing. While Paul traps can now come in many shapes and sizes, the archetype of a so-called macroscopic trap in quantum information processing is still the blade trap (see Fig 1.).
The excellent quantum control that is possible in these naturally identical quantum information carriers, qubits, has allowed our group to spearhead many developments and demonstrations from early breakthroughs such as Cirac-Zoller gate (Nature 422, 408–411 (2003)), or quantum teleportation of the state of an atom (Nature 429, 734–737 (2004)), the first quantum byte (Nature 438, 643–646 (2005)) to recent achievements such as large-scale, full-register, full-connectivity entanglement up to 26 qubits (PRX Quantum 2, 020343 – 2021) or the first universal logical quantum gate set (Nature 605, 675–680 (2022)) for quantum error correction.
  |
| Fig 1.: Left: The ‘Linear trap’ original blade trap in use for two decades. Right: The modern successor trap AQT PINE as used in the AQTION setup. |
Quantum error correction and fault tolerance
The state of quantum systems that may be used for powerful information processing tasks is notoriously fragile. Information we want to store and process is ever at the risk at being entangled with our hot and noisy environment which leads to computational errors. There is no known way to both perfectly isolate quantum information from this detrimental environment while at the same time still being able to manipulate it in any way. Consequently, just like in classical computation, we have to deal with errors. Unfortunately, classical computers are much more resilient against this occurring and the majority of classical error correction schemes are forbidden by the famous no-cloning theorem and collapse of the wavefunction upon measuring.
Fault tolerance and, as its tool, quantum error correction are attempts at still being able to perform arbitrary quantum computation by making use of redundant encoding of information over several physical information carriers.Logical information is stored non-locally such that an error at a single location does not destroy the distributed information, but can be recovered from the ensemble. Quantum error correction based on such collective “undoing” of local errors is difficult and essential. It is therefore considered to be the holy grail of quantum computing. As part of world-wide collaborations our group has been working on experimental error correction protocols and implementations for more than a decade:
Experimental Repetitive Quantum Error Correction Science (2011) Vol 332, Issue 6033 pp. 1059-1061
Quantum computations on a topologically encoded qubit Science (2014) Vol 345, Issue 6194 pp. 302-305
Experimental deterministic correction of qubit loss Nature volume 585, pages 207–210 (2020)
Entangling logical qubits with lattice surgery Nature volume 589, pages 220–224 (2021)
Demonstration of fault-tolerant universal quantum gate operations Nature volume 605, pages 675–680 (2022)
Our recent development focus is now shifting towards larger error correcting codes and performing the first error-corrected quantum algorithms.
Quantum characterisation, verification and validation
The prospect of a quantum computer so powerful that it outperforms even the biggest classical supercomputer is perhaps the greatest motivation for the development of quantum information processors. However, the question arises of how we make sure that the output of such a powerful machine is actually correct once they can no longer be checked by classical computers. How can we trust a powerful quantum machine to not be plain wrong or even maliciously manipulated when we cannot check the result anymore? In quantum characterisation, verification and validation (QCVV) our group has been working on routines and techniques which are supposed to achieve just this task for example by posing the same question to many machines and cross-checking (Phys. Rev. X 11, 031049 – 2021), demonstrating efficient tomographic methods (PRX Quantum 3, 040310 – 2022), or by using post-quantum secure classical means (arXiv:2203.07395 [quant-ph]).
Scalable quantum information processor development
The prospect of working with single quantum systems such as individual atoms and ions was something that the forefathers of quantum mechanics found exceedingly unlikely. However, today control over individual quantum systems is well established. For useful quantum information processing we require many such carriers of quantum information. However, larger registers bring new challenges that are not present when dealing with small numbers of quantum systems. Indeed, the very same reason why quantum computers are potentially powerful makes it also challenging to build larger ones. If the effort we need to expend to operate a quantum information processor grows just as quickly as its computational potential then we cannot reach a regime where they will outperform classical machines. That is to say we cannot need a super-classical machine to operate a super-classical machine. Keeping the resources needed to increase the computational power low is what is frequently referred to as ‘scalability’.
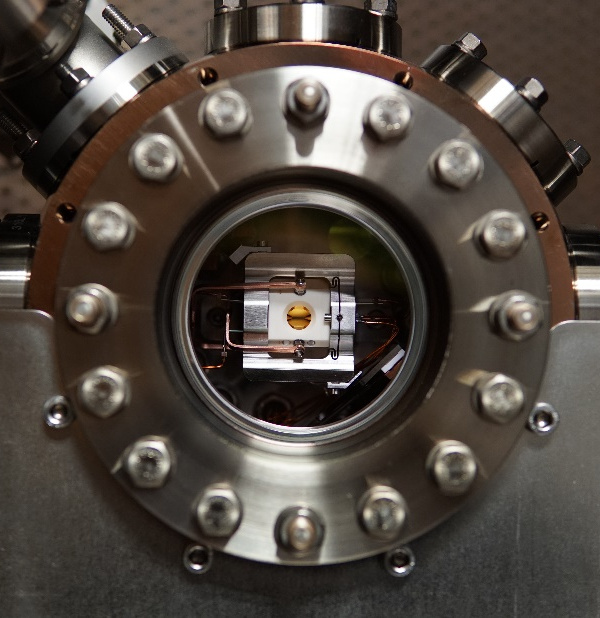
 |
| Fig. 2: Segmented trap in Cryostat |
It is at this point fairly clear that linear ion chains stored in a single potential well as registers are not scalable. For one, if we aim for millions of qubits, that would require a vacuum chamber much larger than any reasonable person would want to have. Scalable trap architectures, their development and figuring out the ins and outs of how to use them is therefore an integral part of learning how to scale up quantum computers. Micro-structured, segmented traps and 2D trap structures are being investigated in our group in multiple setups (see Fig. 2). In such structured traps new operations are both possible and necessary for novel computational paradigms, which require excellent control over the motion of individual ions or subgroups of them. These operations include shuttling of ions, as well as splitting and merging of subgroups, or swapping of places between individual ions.
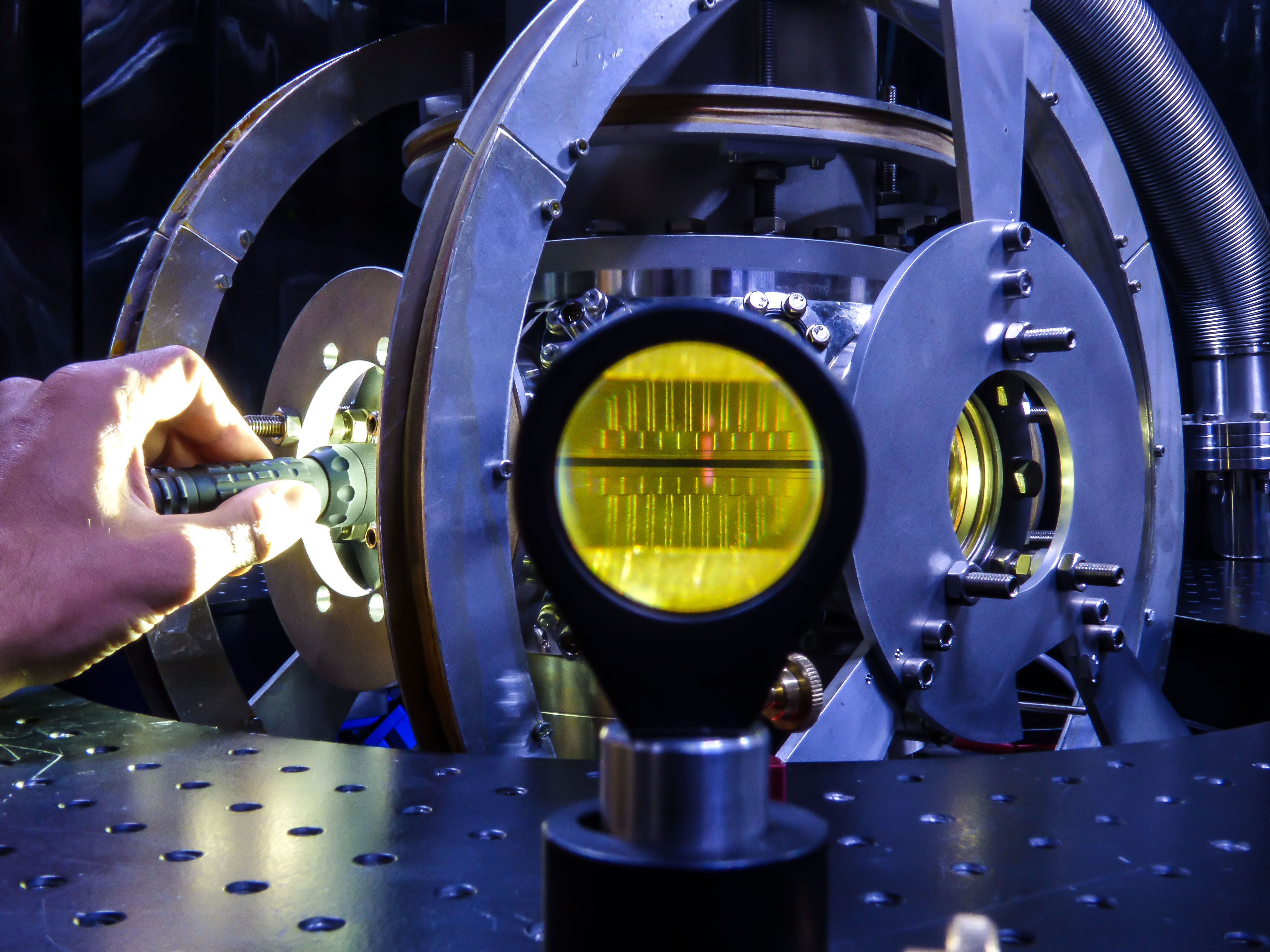
 |
| Fig 3. Quantum Computer in 19'' Rack |
Scalable quantum information processors are a major research direction around the world. However, it is often the case that only individual parts or components of this large-scale endeavour are investigated by research groups. Our group has been part of the AQTION collaboration to figure out what exactly it takes to make a larger quantum processor work. Integrating all components and making them all work together in tandem, reliably, turns out to be a huge task. This is in part due to the fact that routines or techniques that work fine for smaller registers become absolutely unmanageable once register sizes grow past 10 or so qubits.
The 19’’-rack-based quantum computer developed and deployed within AQTION (see Fig 3.) has taken a big step towards realizing quantum computers that can operate reliably with large registers with form factor and operating conditions compatible with computing centres of classical computers (PRX Quantum 2, 020343 – 2021).
With development steadily progressing we are moving forward to the next generation of experimental setups. Gate errors, chiefly entangling gate errors, are still the dominant error source in quantum computers. In the Hyperion experiment we will be focusing on improving existing entangling gate schemes and developing and testing novel schemes to reach fundamental gate infidelities in the 10 parts-per-million range for single qubit, and 100 parts-per-million for entangling operations. Part of this will be achieved by switching from Calcium-40 to Barium isotopes such as Barium-137 which offers some key advantages over Calcium. Not the least of which is that the laser wavelengths required to interact with Barium offer themselves more readily to integrate optics directly into the ion traps to further drive scalability and stability. Moving to an isotope with nuclear spin I = 3/2 further offers, in contrast to Calcium-40, the option to use very stable hyperfine groundstate qubits.
The Quantum Information team is always looking for master’s, PhD students and postdocs! Do not hesitate to inquire with our principal investigator, Thomas Monz (
Project Members

- Florian Kofler (Master Student)
- Tommaso Faorlin (PhD student)
- Lukas Gerster (PhD student)
- Manuel John (PhD student)
- Michael Meth (PhD student)
- Ivan Pogorelov (PhD student)
- Lukas Postler (PhD student)
- Lorenz Panzl (PhD student)
- Roman Stricker (PhD student)
- Peter Tirler (PhD student)
- Claire Edmunds (Postdoc)
- Christian Marciniak (Postdoc)
- Martin Ringbauer (senior scientist)
- Thomas Monz (senior scientist)
- Philipp Schindler (senior scientist)
- Rainer Blatt (group leader)
Former members: Julio Barreiro, Michael Chwalla, Stefan Quint, Rafael Rothganger de Paiva, Virgile Andreani, Anton Nolf, Daniel Nigg, Matthias Brandl, Esteban Martinez, Julian Rickert, Marc Bussjäger, Pavel Hrmo, Thomas Feldker, Alexander Erhard, Verena Podlesnic, Benjamin Wilhelm, Markus Teller, Martin van Mourik

